Compund interest formula

Compound Interest Formula - Explained

Since I launched my compound interest calculator, I have regularly been the recipient of emails asking me to explain the formula for calculating compound interest. For that reason, we're going to tackle this subject today.

The concept of compound interest is that interest is added back to the principal sum so that interest is earned on that added interest during the next compounding period. If you would like more information on what compound interest is, please see the article what is compound interest?. For now, let's look at the formula and go through an example.

Annual compound interest formula

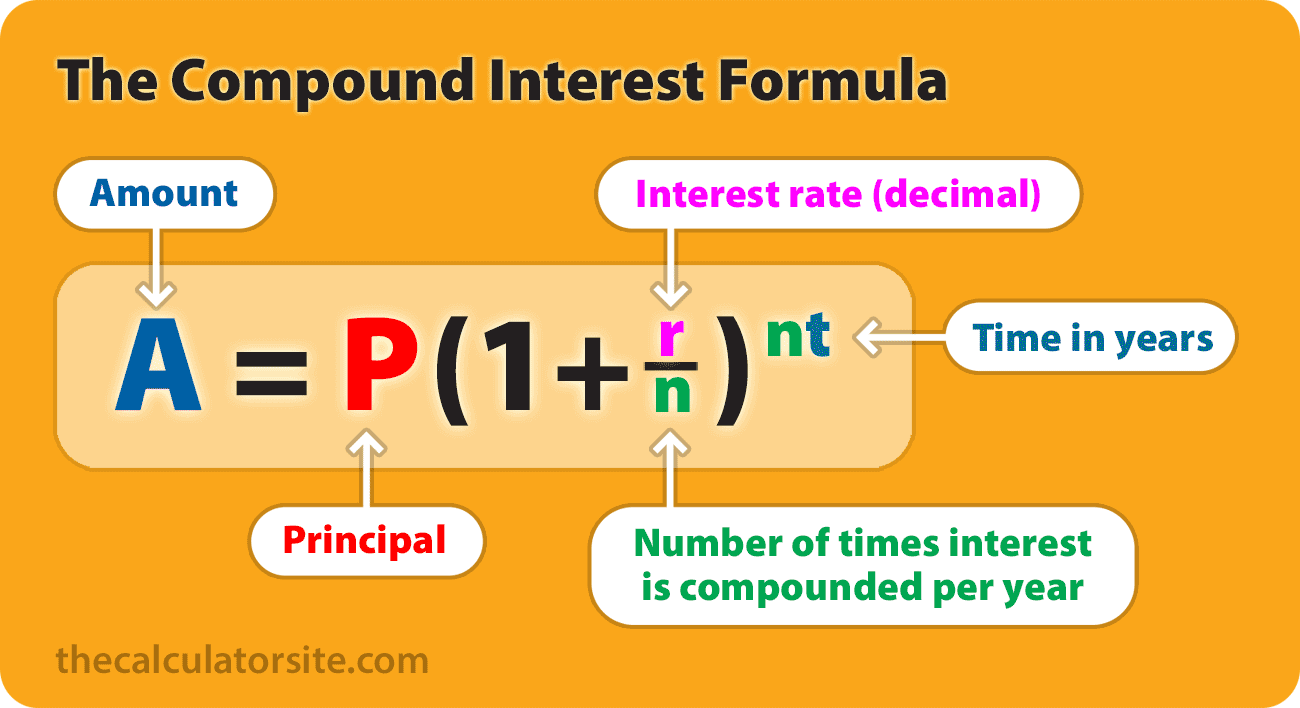
The formula for annual compound interest, including principal sum, is:
A = P (1 + r/n) ^(nt)

Where:

A = the future value of the investment/loan, including interest
P = the principal investment amount (the initial deposit or loan amount)
r = the annual interest rate (decimal)
n = the number of times that interest is compounded per year
t = the number of years the money is invested or borrowed for

Note that this formula gives you the future value of an investment or loan, which is compound interest plus the principal. Should you wish to calculate the compound interest only, you need this:

Total compounded interest = P (1 + r/n) ^(nt)- P

Let's look at an example

Compound interest formula (including principal):

A = P(1+r/n)^(nt)

If an amount of $5,000 is deposited into a savingsaccount at an annual interest rate of 5%, compounded monthly, the value of the investment after 10 years can be calculated as follows...

P = 5000. r = 5/100 = 0.05 (decimal). n = 12. t = 10.

If we plug those figures into the formula, we get the following (note that ^ indicates 'to the power of'):

A = 5000 (1 + 0.05 / 12) ^ (12(10)) = 8235.05.

So, the investment balance after 10 years is $8,235.05.

Methodology

A few people have written to me asking me to explain step-by-step how we get the 8235.05. This all revolves around PEMDAS (also known as BODMAS in the UK) and the order of operations. Let's go through it:

A = 5000 (1 + 0.05 / 12) ^ (12(10))

(note that ^ means 'to the power of')

Using the order of operations we work out the totals in the brackets first. Within the first set of brackets, you need to do the division first and then the addition (division and multiplication should be carried out before addition and subtraction). We can also work out the 12(10). This gives us...

A = 5000 (1 + 0.00416) ^ 120

(note that the over-line in the calculation signifies a decimal that repeats to infinity. So, 0.00416666666...)

Then:

A = 5000 (1.00416) ^ 120

The exponent goes next. So, we calculate (1.00416) ^ 120.

This means we end up with:

5000 × 1.6470095042509848

= 8235.0475.

You may have seen some examples giving a formula of A = P ( 1+r ) ^ t . This simplified formula assumes that interest is compounded once per period, rather than multiple times per period.

The benefit of compound interest

The full benefit of compound interest will become clear when I tell you that without it, your investment balance in the above example would be only $7,500 ($250 per year for 10 years, plus the original $5000) by the end of the term.

So, thanks to the wonder of compound interest, you will gain an additional $735.05.

Interactive compound interest formula

Use the calculator below to show the formula and resulting compound interest calculation for your chosen figures. Note that this calculator requires JavaScript to be enabled in your browser.

Principal amount (P):

Annual rate (r):

  decimal  % 

Compounds per year (n):

Years (t):

calculate

P(1 +rn)(nt)=A

For a comprehensive set of tools for calculating compound interest on your savings, please see our compound interest calculators.

On the next page we look at the formula for compound interest with monthly contributions (how you can add a regular, additional monthly deposit) and the future value formula.

1

2

NEXT PAGE ▶

 

          

696 votes

Last